“I do not believe that historical data from the WSPR network can provide any information useful for aircraft tracking.”
Prof. Joe Taylor (K1JT), Nobel Prize in Physics, Inventor of WSPR
Despite many stories in the media repeating claims that historical WSPR data can be used to track MH370, there are many reasons why these claims are patently false. There is broad agreement among knowledgeable researchers that have investigated these claims, and a handful of these researchers have documented their concerns. For instance, amateur radio enthusiast Hayden Haywood (VK7HH) has created a video explaining why, in simple terms, WSPR can’t track airplanes. MH370 investigator Steve Kent published a paper that formally treats skywave propagation and scatter off airplanes, and concludes there is insufficient signal strength (by many orders of magnitude) for WSPR to detect aircraft over long distances. In fact, even WSPR creator Joe Taylor (K1JT), who won a Nobel prize in physics for his research on pulsars and gravity, told fellow MH370 Independent Group (IG) member Mike Exner, “I do not believe that historical data from the WSPR network can provide any information useful for aircraft tracking.”
WSPR Background
WSPR (pronounced “whisper”) is an acronym for “Weak Signal Propagation Reporter”. Amateur radio stations implementing WSPR send and receive messages using low-power transmissions to test propagation paths on the Low Frequency (LF), Medium Frequency (MF), High Frequency (HF), and Very High Frequency (VHF) amateur radio bands. When a participating station successfully decodes the transmission transmitted by another participating station, it sends that information to a central database, and that information is available to the public for retrieval. For each 110-second contact between stations (“spots”), the available information includes station call signs, locations, transmitted power, and three parameters discriminated by the receiver: signal-to-noise ratio (S/N), frequency, and frequency drift. The proposed theory is that recorded deviations (“anomalies”) in the (S/N) and the frequency shifts/drifts are related to radio wave interactions with aircraft some thousands of kilometers distant from either amateur radio station.
The theory behind using bi-static radar (i.e., transmitter and receiver in different locations) for aircraft detection and tracking is well-known, and books (e.g., this) have been written on this subject. A special case is when an aircraft crosses the “baseline” between transmitter and receiver, resulting in a “forward scattered” signal caused by the diffraction around the silhouette (projected area) of the aircraft. The Forward Scatter Radar Cross Section (FSRCS) is typically much larger than the RCS that conventional mono-static radar uses to detect targets. It is this forward scattered signal that is of interest here in evaluating whether WSPR signals can be used to reconstruct the path of MH370.
In this article we apply the well-developed theory of bi-static radar to demonstrate that WSPR signals cannot be used to detect MH370 in the manner claimed in this paper. The relevant equations are presented in the Appendix, and the inputs and the calculational results for the test cases can be found in the accompanying table in the Appendix.
Detection of MH370 Before Radar Coverage Was Lost
We consider the claim that the WSPR data shows that MH370 was detected on the night of the disappearance at 17:16 UTC when it was still under radar coverage as it flew over the Gulf of Thailand towards waypoint IGARI, before the turnback, at FL350 (37,200 ft). At that time, a WSPR transmission from a station in Switzerland (HB9CZF) was received by an Australian station near Canberra (VK1CH) over a distance of 16,527 km on 14.097 MHz at a transmitted power of 1 W. The distance from the Swiss transmitter to the aircraft was 9,868 km and the distance from the aircraft to the receiver was 6,660 km, as depicted in this figure from the paper:
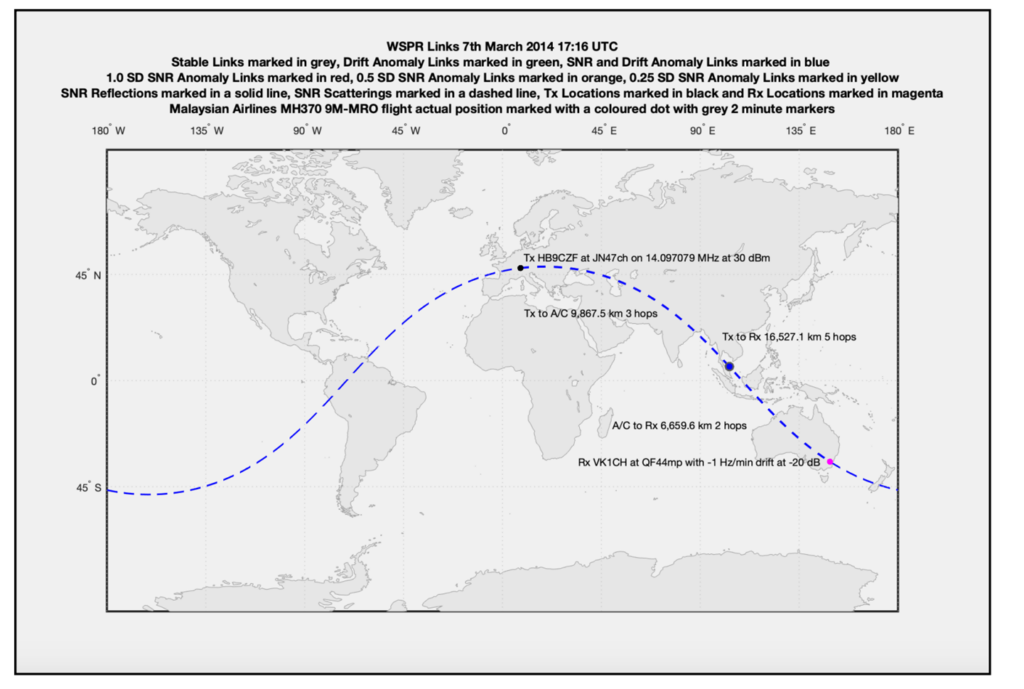
Although WSPR contacts greater than 16,000 km are rare, this spot shows they can indeed occur. Based on the distance between the stations, the transmission from Switzerland reached the Australian station via skywave propagation in which the radio waves were refracted off the ionosphere and reflected off the Earth’s surface (“hops”) about 5 times.
WSPR Signals Forward Scattered from an Aircraft Would be Undetectable at Long Distances
The column labeled “Case 1” from table in the Appendix shows the inputs and the calculational results for this scenario. Assuming that the propagation loss is the same as for free-space propagation, the expected strength of the direct signal at the receiver is -110 dBm, which is about the same value claimed in the paper when considering hops between the ionosphere and the Earth’s surface. This suggests that the refraction and reflection losses were either calculated to be very small, or were neglected.
At 14.097 MHz, the wavelength is 21.3 m, and the FSRCS for the B777-200ER for waves directly incident to the top or bottom is estimated to be 18,791 m2, or about 23 times the projected area. The forward scattered signal at the receiver is estimated to be -210 dBm, or about 100 dB (10 orders of magnitude) weaker than the direct signal. Can a signal of this strength be detected and decoded by the WSPR software?
Whether the signal could be detected by the radio and decoded by the software depends on the achievable noise level, as a minimum signal-to-noise ratio (S/N) of around -30 db is required by the WSPR software, where the noise level is referenced to a bandwidth of 2.5 kHz. I ran some simple experiments on my Flex 6400 amateur radio to measure the achievable noise level on the 20-meter band at my home in suburban Roanoke, Virginia. At 10:30 am on December 19, 2021, on a quiet part of the band, when connected to a horizontal resonant antenna, and after setting the bandwidth to 2.5 kHz, I measured a noise floor of -102 dBm. This largely consists of manmade and natural noise received at the antenna. To determine the sensitivity of the radio independent of the environmental noise, I disconnected the antenna and connected the radio to a resistive dummy load of 50 ohms. The noise level dropped to -105 dBm. By using the radio’s built-in pre-amplifier configured for its maximum gain of 32 dB, the noise level further dropped to -129 dBm. (Pre-amplification improves sensitivity but increases the distortion from strong signals, and so must be used judiciously.) Even though this noise level would be very difficult to achieve under real conditions, I used this noise level as the reference for calculating (S/N) values on 20 meters.
So, for the forward scattered signal strength of -210 dBm, the (S/N) would be (-210 – -129) = -81 dB. This is 51 dB weaker than WSPR requires (-30 dB), i.e., the forward scattered signal is 5 orders of magnitude too weak to be detected and decoded by WSPR! Considering the very favorable assumptions we made regarding propagation loss, incident angle, and noise floor, we can be quite confident that the WSPR signal originating in Switzerland at 17:16 UTC did not interact with MH370 in any way that was detectable in Australia, as was claimed.
WSPR Signals Forward Scattered by an Aircraft Would Be Masked by the Stronger Direct Signal
Assuming the skywave propagation loss was equal to the free-space propagation loss, the WSPR signals originating in Switzerland and forward scattered by MH370 over the Gulf of Thailand would be received in Australia with a strength of around -210 dBm. However, the direct radio waves that did not interact with the aircraft would be received with a strength of around -110 dBm. That means that the direct signal strength would be about (-110 – -210) = 100 db (10 orders of magnitude!) stronger than the scattered signal. Under these circumstances, the combined signal (direct plus forward scattered) would be absolutely indistinguishable from the direct signal, even if above the noise level (which it was not).
However, the equations presented in Appendix A predict that it IS possible for radio waves to forward scatter from an aircraft and be detected under the right conditions. For example, Joki et al. studied how broadcast TV transmissions at around 50 MHz may be passively used to detect, identify, and track airliners over a distance of hundreds of kilometers. Some of the factors that determine whether the aircraft could be detected include:
- The projected area of the aircraft
- Strength of the direct signal received, i.e., high power transmitters near the receiver increase the signal strength
- The distance of the aircraft to the receiver, i.e., the proximity of the aircraft increases the strength of the forward scattered signal
- The frequency of the transmission, higher frequencies increase the FSRCS and therefore the strength of the forward scattered signal
- Frequency-based signal processing to separate the direct signal from the Doppler-shifted forward scattered signal
Recently, amateur radio operator Nils Schiffhauer (DK8OK) claims to have observed aircraft scatter by analyzing the signal from an AM broadcast of China Radio International (CRI), which operates on 17.530 MHz with a 250 kW carrier, and uses a beam antenna with a gain of 25 dBi towards Europe. Nils’ location is near Hannover Airport in Germany, some 7,600 km away from the CRI transmitter in Xianyang, China. The figure below depicts a “waterfall” plot showing aircraft scatter over a period of 3 hours. The Doppler-shifted signals from many aircraft are clearly visible in the lower sideband (LSB), some 5 to 20 Hz below the carrier frequency.

After processing the data from a 1-hour measurement, Nils calculated that the carrier strength was -59.1 dBm, the average Doppler signal strength was -105.9 dBm, and the average noise level was -108 dBm.
I was curious if the forward scatter equations in Appendix A would produce calculational results consistent with Nils’ measurements. After using FlightRadar24 to observe flights passing near his residence, I estimated that planes landing on Hannover Airport’s Runway 27R would generally pass within a lateral distance of about 2 km and about 0.85 km (2800 ft) above his residence, which is a slant range of about 2.2 km . A good number of those airplanes were B737s, which I used to calculate the FSRCS. The inputs and the results from the calculations are shown in the column labeled “Case 2” from the table in Appendix.
We know the location, power, and antenna gain of the transmitter, and since we know the received strength of the carrier was -59 dBm, we can calculate the additional propagational loss of the skywave path due to refractions from the ionosphere and reflections from the Earth’s surface, which we estimate to be around -33 dB. The signal strength of the aircraft scatter is then calculated to be around -102 dBm, which is only 4 dB stronger than the measured value of -106 dBm. Considering that the value of FSRCS is assumed to be in the most favorable direction, the measured strength of the aircraft scatter is entirely consistent with the calculated value.
Nils concludes that since the signal from the aircraft scatter is 47 dB below the carrier, it would be impossible to look at the combined signal (which is all that is available in the WSPR database) and determine the contribution of the aircraft scatter. We strongly agree.
WSPR Signal Deviations are Not Related to Aircraft
Based on the extremely small signal generated by a hypothetical interaction with MH370 at 17:16 UTC, there can be little doubt that at that time, the WSPR database did not record a spot between Swiss and Australian stations consistent with forward scatter from the aircraft.
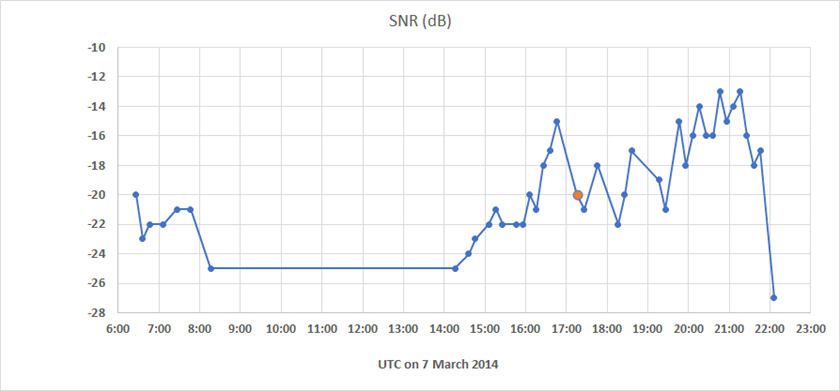
Yet it’s claimed that there was a detectable deviation in the recorded (S/N) values between the Swiss and Australian stations that is indicative of forward scatter from MH370. To evaluate this claim, Mike Exner and Bobby Ulich produced the following graph which shows the (S/N) for all WSPR contacts between the Swiss (HB9CZF) and Australian (VK1CH) stations over an time interval of around 16 hours. The particular (red) spot deemed as “anomalous” clearly shows no greater deviation from the trend than any other spot. What is claimed to be “anomalous” is within the scatter range of the other points. The dynamic characteristic of the ionosphere is all that is needed to explain these deviations.
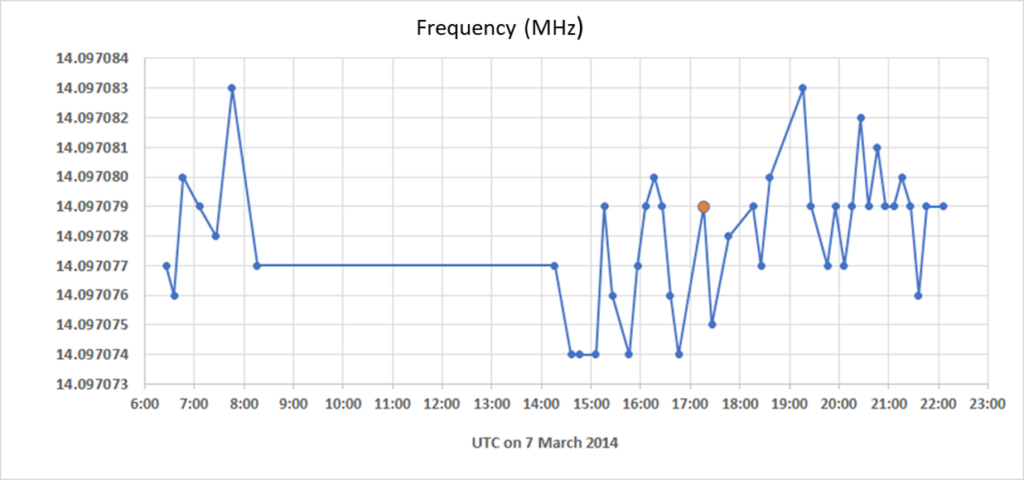
To further demonstrate that there is nothing anomalous about the spot at 17:16 UTC, Mike and Bobby produced the following graphs which show that the reported values of frequency and frequency drift at 17:16 UTC are in no way anomalous to the other values recorded on that day for HB9CZF-VK1CH WSPR contacts.
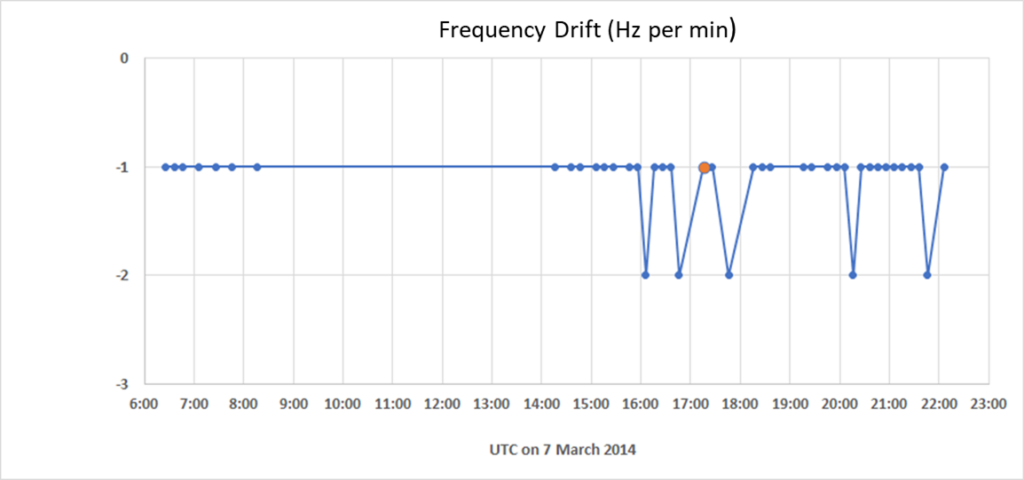
Conclusions
This article attempts to lay out in simple technical terms why WSPR data cannot be used to track aircraft over long distances, and certainly cannot be used to reconstruct the flight path of MH370. At long distances and at low transmission powers, the received signals from hypothetical aircraft scatter are simply too weak by many orders of magnitude. What is claimed to be discernable “anomalies” in signal strength attributable to forward scatter by aircraft are within the expected deviations in signal strength for long distance skywave propagation involving refraction off the ionosphere. Although aircraft scatter could be detected if the aircraft were close to either the transmitter or receiver and if the transmitted power were sufficiently strong, the detection of the aircraft requires signal processing to separate the Doppler-shifted scattered signal from the much stronger direct signal, and this data is not available in the WSPR database.
Acknowledgements
This article benefited from many private communications with Mike Exner, Don Thompson, Bobby Ulich, Steve Kent, Nils Schiffhauer, John Moore, and Ed Anderson. I also acknowledge the many insightful blog comments from Mick Gilbert, Sid Bennett, and @George G.
Update on December 22, 2021
I asked Joe Taylor for a comment on the material covered in this article. Here was his response, shared with his permission:
As I’ve written several times before, it’s crazy to think that historical WSPR data could be used to track the course of ill-fated flight MH370. Or, for that matter, any other aircraft flight…
I don’t choose to waste my time arguing with pseudo-scientists who don’t understand what they are doing.
Appendix: Equations and Table of Results

where the variable definitions and the inputs and results for the two cases can be found in the table below:

Reference: Passive Radar Technology, Prof. Christopher Baker, University of Birmingham, NATO STO-EN-243-02.